Definition:
Equivalent fluid pressure is a simplification of backfill earth pressure against retaining structures. If the backfill behind a cantilever retaining wall or restrained (basement) wall is level, we can use the equivalent pressure. For a cantilever retaining wall, level & drained condition (subdrain), the equivalent fluid pressure is simply the product of Ka * Gamma, where Ka is the Active earth pressure coefficient & Gamma is the moist unit weight of the backfill. For a restrained retaining wall, level & drained condition (subdrain), the equivalent fluid pressure is simply the product of Ko * Gamma, where Ko is the At Rest earth pressure coefficient & Gamma is the moist unit weight of the backfill.
History:
The first mention of the equivalent fluid pressure is in the 1953 publication, “American Association of State Highway Officials, Standard Specifications for Highway Bridges”, currently known as AASHTO Specifications. It said “Structures designed to retain fills should be proportioned to withstand pressure as given by Rankine’s formula; provided, however, that no structures should be designed for less than an equivalent fluid pressure of 30 lb per cu ft”.
Prior discouragement of the EFP method:
Professor Whitney Clark Huntington in his publication, “Earth Pressures and Retaining Walls”, said “The equivalent fluid method is commonly misused by applying it to walls which retain earth with a sloping ground surface..”. This statement was made in 1957, approx. 60 years ago. However, we still see the use of EFP method in today’s retaining wall designs.
Why is EFP not applicable to ascending slopes?
- The EFP is increased arbitrarily to account for the ascending slope backfill angle while the vertical earth pressure component is sometimes not accounted for in design
- The direction of the static earth pressure is level but in reality should be parallel to the ascending slope when using the Rankine’s method
- The direction of the static earth pressure is level but in reality should be same as the soil-wall friction angle in Coulomb’s method
- The sliding factor of safety computed by EFP will be larger than that calculated from Rankine, Coulomb or Muller-Breslau method (unconservative).
- The overturning factor of safety computed by EFP will be smaller than that calculated from Rankine, Coulomb or Muller-Breslau method (overly conservative).
- The Toe bearing pressure will be smaller with EFP method as compared to Rankine, Coulomb or Muller-Breslau method (under-predict induced wall settlement)
- The Heel bearing pressure will show higher value with the EFP method as compared to Rankine, Coulomb or Muller-Breslau method (in reality eccentricity >B/6)
- The Moment Demand, Mu, will be slightly larger with the EFP method as compared to Rankine, Coulomb or Muller-Breslau method (extra stem steel required)
- The top of wall deflection will be slightly larger with the EFP method as compared to Rankine, Coulomb or Muller-Breslau method
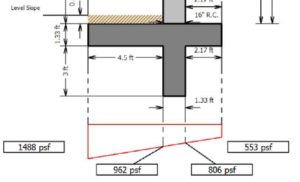 Bearing Press.(EFP method)
Bearing Press.(EFP method) 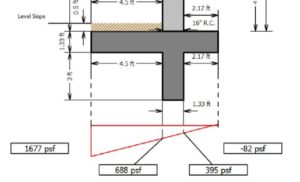 Bearing Press.(Phi/C /Gamma method)
Bearing Press.(Phi/C /Gamma method)
Conclusion:
Geotechnical engineers should start to offer Phi, C, and Gamma values for cantilever and restrained retaining wall design & Wall designers should learn how to compute Active Ka, At Rest Ko, Passive resistance Kp, and Sliding friction coefficient. Professor Huntington discouraged it 70 years ago and SoilStructure Software discourages its use today. EFP is still in v3 of the SoilStructure Retaining Wall software as we hope this is a transitional time from EFP to Phi/C/Gamma.
